We’ve already analyzed tens of thousands of financial research papers and identified more than 700 attractive trading systems together with hundreds of related academic papers.
Browse Strategies- Unlocked Screener & 300+ Advanced Charts
- 700+ uncommon trading strategy ideas
- New strategies on a bi-weekly basis
- 2000+ links to academic research papers
- 500+ out-of-sample backtests
- Design multi-factor multi-asset portfolios
Upgrade subscription
The implied volatility from stock options is usually bigger than the actual historical volatility. The research, therefore, suggests the possibility to earn a systematic risk premium by selling at-the-money options short-term. Numerous papers show that this premium is quite substantial – selling put options gives average returns ranging from 0.5% to 1.5% per day.
However, strong caution is needed in implementing these short volatility strategies (strategies that exploit the volatility premium by selling volatility – usually selling put options or straddles) as the return distribution is very abnormal (put sellers historically could incur losses up to -800%). There is also a strong serial correlation in large negative days (from the put seller’s point of view); therefore, substantial margin reserves are needed when implementing these strategies, and returns are then much lower. We present a simple options strategy exploiting the option premium, with a backtested period, which includes the 1987 crash.
Fundamental reason
Most researchers speculate that the volatility premium is caused by investors who strongly dislike negative returns and the high volatility on equity indexes and are therefore willing to pay a premium for portfolio insurance offered by puts.
Other researchers explain the volatility premium with the Peso problem (Black Swan event) – a situation when a rare but influential event could have reasonably happened (and removed the premium) but did not happen in the sample; this explanation is, however, highly unlikely as other researchers show that huge market crashes would have to occur every few years to remove the volatility premium altogether.
- Unlocked Screener & 300+ Advanced Charts
- 700+ uncommon trading strategy ideas
- New strategies on a bi-weekly basis
- 2000+ links to academic research papers
- 500+ out-of-sample backtests
- Design multi-factor multi-asset portfolios
Backtest period from source paper
1986-1995
Confidence in anomaly's validity
Strong
Indicative Performance
26%
Notes to Confidence in Anomaly's Validity
Notes to Indicative Performance
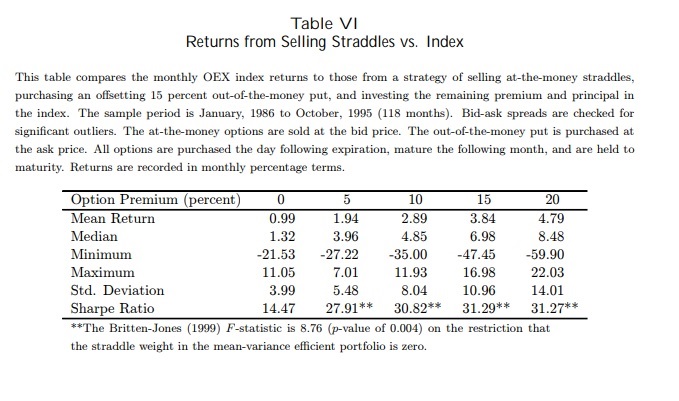
per annum, annualized (geometrically) monthly performance 1,94% from table VI
Period of Rebalancing
Monthly
Notes to Period of Rebalancing
Notes to Estimated Volatility
Number of Traded Instruments
4
Notes to Number of Traded Instruments
Notes to Maximum drawdown
Complexity Evaluation
Moderately complex strategy
Notes to Complexity Evaluation
Financial instruments
futures, options, swaps
Simple trading strategy
Each month, at-the-money straddle, with one month until maturity, is sold at the bid price with a 5% option premium, and an offsetting 15% out-of-the-money puts are bought (at the ask price) as insurance against a market crash. The remaining cash and received option premium are invested in the index. The strategy is rebalanced monthly.
Hedge for stocks during bear markets
No - Absolutely not a hedge, volatility risk premium strategy is a fat-tail strategy that loses a lot of money during crisis periods…
Out-of-sample strategy's implementation/validation in QuantConnect's framework
(chart+statistics+code)