It Is Hard to Profit by Buying Options and Betting on Higher Volatility
A new financial research paper has been published and is related to:
#20 – Volatility Risk Premium Effect
Authors: Israelov, Tummala
Title: Being Right is Not Enough: Buying Options to Bet on Higher Realized Volatility
Link: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=3248500
Abstract:
Speculators who wish to bet on higher future volatility often purchase options to “go long volatility.” Should investors who buy options expect to profit when realized volatility increases? If so, under what conditions? To answer these questions, we conduct an analysis of the relationship between long volatility performance (buying options) and contemporaneous changes in volatility. We find that buying one-month S&P 500 options is only consistently profitable in the highest decile of changes in one-month volatility. Buying options consistently loses money in the lowest seven deciles of changes in volatility. We then study the trade entry and exit timing required to retain the profits from long option positions during significant volatility increases. We find similar results in global equity option markets.
Notable quotations from the academic research paper:
"During most of the mid-2010s, the S&P 500 Index’s volatility hovered near its historical lows. This calm environment has led many investors to ask whether low volatility presents an opportunity to obtain “cheap” portfolio downside protection by purchasing index options. Israelov and Nielsen (2015) show that the answer to this question is no. They show that the Volatility Risk Premium (VRP) also exists in low volatility environments, and therefore that portfolio protection is still expensive even in calm markets.
Portfolio protection, however, is not the only rationale for purchasing options. A long option position allows a speculator to bet on rising volatility. Many proponents of long volatility exposure often advocate this trade in a low implied volatility market environment. Heightened levels of uncertainty, geopolitical risk, or merely a reversion to long-term volatility are some of the arguments put forth by those who expect volatility to spike.
A recommendation to buy options to capture a predicted increase in volatility is predicated on the assumption that investors profit from long options (or long volatility) positions when volatility increases. This paper tests that assumption.
Although it may seem unintuitive, we show that long volatility positions can lose money even when realized volatility rises. How can this be true? Because, long volatility investors must overcome the well-documented VRP – the difference between implied and realized volatility – just to break even. On average this VRP spread is around 3% in the S&P 500. Therefore, the VRP spread typically accrues to the volatility seller, paid by the long volatility investor. As such, long volatility investors enter their trade facing a significant head wind.
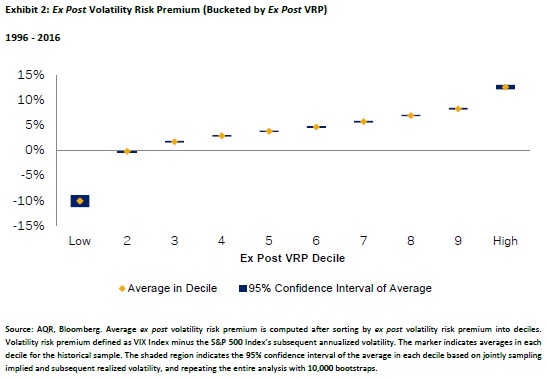
Exhibit 2 sorts the ex post VRP into deciles and reports the average within each decile. On average, equity index options have been richly priced, with an average VRP of 3.0%. The VRP was positive around 85% of the time. Correspondingly, Exhibit 2 shows that, on average, the VRP is positive in eight of the ten deciles, approximately flat in one decile, and negative in one decile. This result implies that long volatility positions in one-month equity index options have been profitable infrequently.
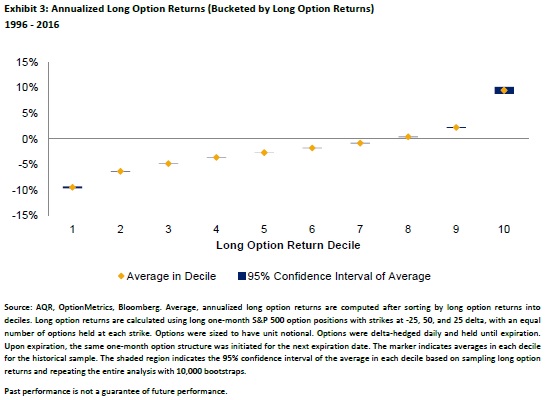
Exhibit 3 sorts the returns of the long option portfolios into deciles, and reports their average annualized return within each decile. Similar to Exhibit 2, Exhibit 3 shows that long option portfolios were infrequently profitable (28% of the time). Average returns were negative in seven of the ten deciles, flat in one decile, and profitable in two. Sorting on actual profitability reveals that there are relatively few profit opportunities.
Volatility mean-reverts over the long run. With equity volatility at historical lows in the mid-2010s, many market commentators suggested long volatility positions in order to bet on increases in volatility. But are bets on increasing volatility actually profitable when volatility increases?
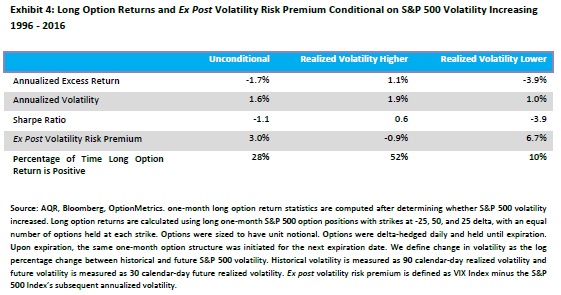
Using our change in volatility measure, we document that over 30-day periods volatility increased 43% of the time in the S&P 500 between 1996 and 2016. Exhibit 4 reports the long option portfolio’s return properties conditional on only holding options during those 30-day periods in which volatility actually increased. For a more complete picture, the exhibit also reports return properties of the unconditional long option portfolio in which the investor does not attempt to time, as well as return properties conditional on periods in which volatility decreased. Having perfect foresight into whether equity markets become more volatile is profitable, but not reliably so. The conditional ex post VRP is -0.9% during periods in which volatility increased versus the 3.0% unconditional VRP. During periods when volatility increased, the average conditional delta-hedged long option annualized return is 1.1%, with a 0.6 Sharpe ratio. This is certainly better than the unconditional long option annualized return of -1.7%, with a -1.1 Sharpe ratio, and considerably better than the conditional long option annualized return of -3.9% and corresponding -3.9 Sharpe ratio during periods when volatility decreased. However, even when realized volatility increases, long option returns are only profitable about half the time. A small set of out-sized volatility increases is what led to positive conditional average long option returns. This suggests that even knowing with certainty that volatility will increase is not enough to reliably profit from a long volatility position. The investor is left with a coin flip in terms of the hit rate of holding a long option position in this scenario.

Then what condition is sufficient to reliably profit? We sort the ex post VRP into deciles by change in volatility and report the average in each decile in Exhibit 5. The ex post VRP is only negative in the tenth decile. It is also interesting that the first decile’s average VRP is more positive than the tenth decile’s is negative.
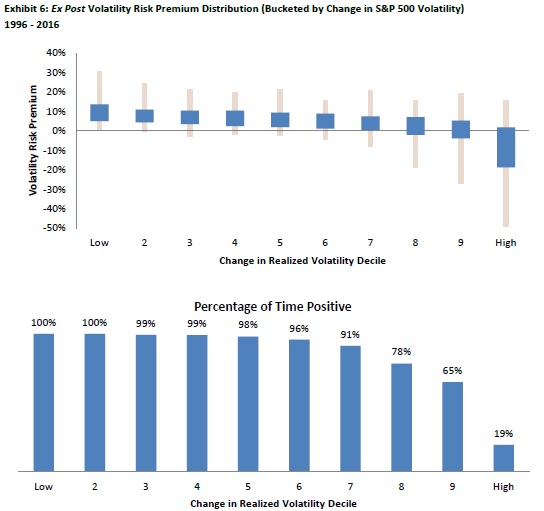
Exhibit 6 shows the distribution of the ex post VRP within each decile. The ex post VRP was positive more than 90% of the time in seven of the ten deciles, and positive more than 65% of the time in the eighth and ninth decile. The only decile in which the ex post VRP was consistently negative was the tenth decile. Correspondingly, large increases in volatility are the only time that we expect long option positions to be profitable.
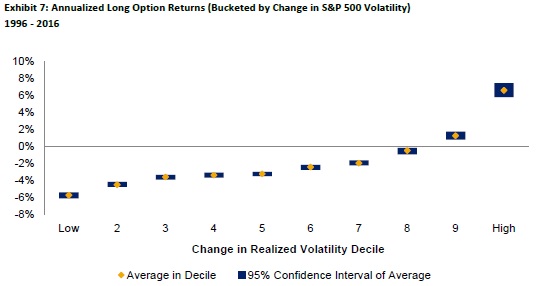
Realized option returns are likely more interesting to option investors than the difference between implied and realized volatility. In that regard, we next sort long option returns into deciles by change in volatility, and report the average annualized return in each decile in Exhibit 7.
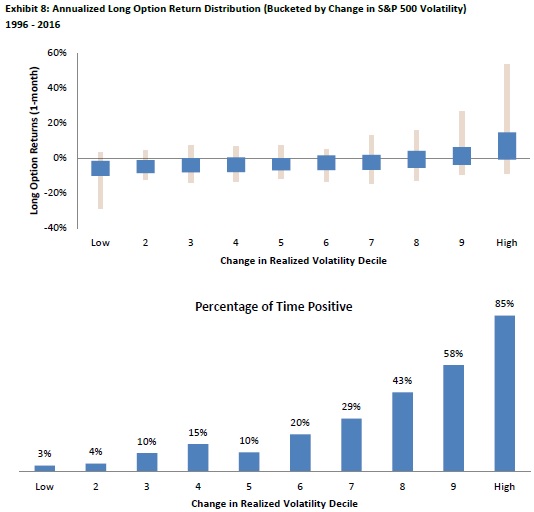
Average long option returns were negative in eight of the ten deciles. Exhibit 8 shows the distribution of long option returns in each decile. Long option returns were consistently negative in most deciles, positive slightly more than half the time in the ninth decile, and consistently positive (85% of the time) in the tenth decile.
Overall, the results for long option returns are similar to those found for the ex post VRP. To consistently profit from a long volatility position on the basis of forecasting changes in volatility, you would have had to predict a 10% probability outcome. The average, annualized long option return in this decile was 6.6% and the average return across the other nine deciles was -2.7%.
Due to these considerations, tactical long volatility traders face a steep uphill battle. Managers who are able to successfully implement this strategy should be given credit for demonstrating exceptional skill (or congratulated for their good luck). However, some amount of healthy skepticism is likely warranted for those who claim to consistently have such exceptional skill."
Are you looking for more strategies to read about? Check http://quantpedia.com/Screener
Do you want to see performance of trading systems we described? Check http://quantpedia.com/Chart/Performance
Do you want to know more about us? Check http://quantpedia.com/Home/About
Follow us on:
Facebook: https://www.facebook.com/quantpedia/
Twitter: https://twitter.com/quantpedia
Share onLinkedInTwitterFacebookRefer to a friend