The Size Effect Has a Lottery-Style Payoff
A new research paper related mainly to: #25 – SIze Premium Authors: McGee, Olmo Title: The Size Premium As a Lottery Link: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=3279645 Abstract: We investigate empirically the dependence of the size effect on the top performing stocks in a cross-section of risky assets separated by industry. We propose a test for a lottery-style factor payoff based on a stochastic utility model for an under-diversified investor. The associated conditional logit model is used to rank different investment portfolios based on size and we assess the robustness of the ranking to the inclusion/exclusion of the best performing stocks in the cross-section. Our results show that the size effect has a lottery-style payoff and is spurious for most industries once we remove the single best returning stock in an industry from the sample each month. Analysis in an asset pricing framework shows that standard asset pricing models fail to correctly specify the size premium on risky assets when industry winners are excluded from the construction of the size factor. Our findings have implications for stock picking, investment management and risk factor analysis. Notable quotations from the academic research paper: ” Firms with small market capitalization tend to outperform larger companies. Investors are attracted to lottery-like assets with positively skewed returns because they o ffer a very large payoff with a small probability, which the investors overweight. This demand makes positively skewed securities overpriced and likely to earn low returns. In this article we test whether the size/market capitalization attribute, and associated factor-mimicking portfolios, receive a lottery-like payoff . The implications of this are that most small stocks do not payoff and the returns to a size strategy are driven by a small number of winners. This type of payo ff can be captured through diversi fication but leaves an under-diversifi ed investor exposed. The risk being that they will not include winning stocks and their resulting return expectation is negative. To investigate the e ffect of winning stocks on the performance of investment portfolios based on the size we propose a conditional logit model for ranking di fferent investment portfolios based on size and assess the robustness of the ranking to the inclusion/exclusion of the best performing stocks in the cross-section. This parametric choice is embedded within a stochastic utility model for explaining the investment decisions of under-diversi fied size investors aiming to exploit the so-called size premium. under-diversifi ed individuals maximize their expected utility in each period by choosing the stock that is predicted to yield the highest return (highest positive skew). This choice is driven by market capitalization of the portfolio and modeled parametrically using the conditional logit model. In order to obtain cross-sectional variation on the relationship between the size e ffect and portfolio performance we split the whole cross-section of stocks into di fferent industries and fi t the conditional logit model to each industry separately. We apply the conditional logit model at an industry-speci fic level across three ranked sorted portfolios based on market capitalization: a small, mid-size and big portfolio created from the stocks in each tercile of the cross-section of assets in a speci fic industry ranked by asset size. This exercise is repeated for 20 industries over the period January 1970 to November 2015. Our results reveal that the size e ffect vanishes once the top performing stocks in an industry are removed from the sample.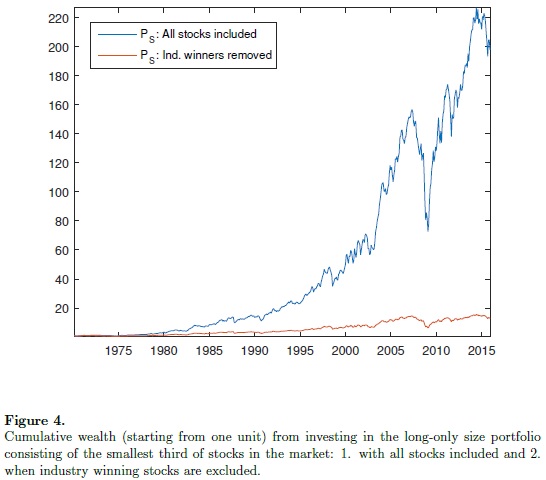
| Do you want to test these ideas yourself? We offer our readers Historical Trading Data Discounts. |
Are you looking for more strategies to read about? Sign up for our newsletter or visit our Blog or Screener.
Do you want to learn more about Quantpedia Premium service? Check how Quantpedia works, our mission and Premium pricing offer.
Do you want to learn more about Quantpedia Pro service? Check its description, watch videos, review reporting capabilities and visit our pricing offer.
Are you looking for historical data or backtesting platforms? Check our list of Algo Trading Discounts.
Would you like free access to our services? Then, open an account with Lightspeed and enjoy one year of Quantpedia Premium at no cost.
Or follow us on:
Facebook Group, Facebook Page, Twitter, Linkedin, Medium or Youtube
Share onLinkedInTwitterFacebookRefer to a friend