Authors: Blitz, Vidojevic

Title: The Characteristics of Factor Investing

Link: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=3206798

Abstract:

We dissect the performance of factor-based equity portfolios using a characteristics-based multi-factor expected return model. We show that generic single-factor portfolios, which invest in stocks with high scores on one particular factor, are sub-optimal, because they ignore the possibility that these stocks may be unattractive from the perspective of other factors. We also show that differences in performance between (i) integrated and mixed-sleeve multi-factor portfolios, (ii) small-cap and large-cap factor portfolios, and (iii) equal and value-weighted factor portfolios can be fully attributed to the differences in their factor characteristics. We conclude that efficient factor investing requires a recognition and understanding of how factor characteristics drive portfolio returns.

Notable quotations from the academic research paper:

"We show that generic single-factor portfolios, which are strategies that invest in stocks which score highly on one particular factor are generally sub-optimal, because they ignore the possibility that these stocks may be unattractive from the perspective of other factors. The negative contributions from other factors cause these strategies to have a substantial weight in stocks with negative expected and ex-post realized market-relative returns. We also show that some stocks have such poor factor characteristics that their expected returns end up being lower than returns on Treasuries. By simply removing those stocks from the market portfolio ex-ante, the realized market return increases by 16%, in relative terms, over the sample period that spans more than five decades.

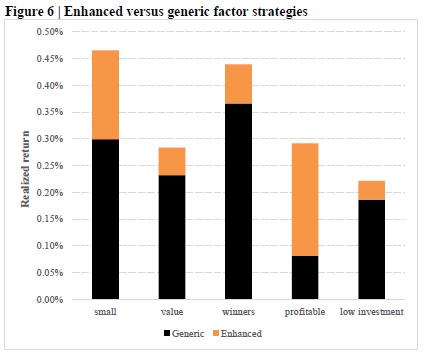
We examine what happens to performance if, each month, we simply remove stocks that have a negative predicted market-relative return from generic factor portfolios. The performance of such enhanced factor strategies is shown in Figure 6. Compared to the generic factor strategies from which they are derived, the performance improvements are about 20% for the value, momentum and investment strategies and about 50% for the small-cap strategy. For the profitability strategy, performance more than triples, from 0.08% to 0.29% per month. This large improvement is not surprising, because a much bigger adjustment is made to this portfolio than to the other factor portfolios. These results imply that generic factor strategies are sub-optimal, and that, even when targeting one particular factor premium, investors should not ignore other established factor premiums.

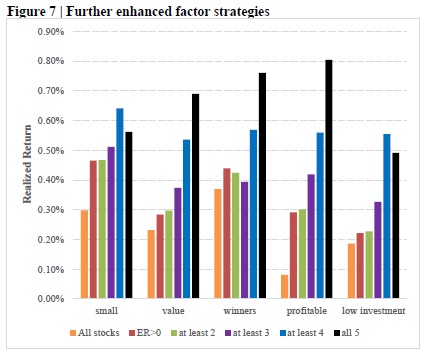
Excluding stocks with expected underperformance helps to enhance a single-factor strategy, but the resulting portfolios can still have stocks with negative exposures to other factors that detract from their performance. We next examine how performance changes if in addition to removing stocks with expected underperformance, we also require stocks to have a non-negative exposure (z-score) to at least one, two, three or four other, non-targeted premiums. Figure 7 shows that realized, full-sample returns of each single factor strategy tend to increase as we require stocks in the portfolios to have non-negative exposures to more factors. For instance, our raw value strategy has a return of 0.23% a month, which increases to 0.28% per month if we ex-ante exclude stocks with negative expected excess returns. If in addition, at the time of portfolio formation, we require that stocks have non-negative exposures to at least two, three, four, and all five factor premiums, the strategy returns increase to 0.30%, 0.37%, 0.54%, and 0.69%, respectively. The number of stocks in the portfolio decreases as we impose more constraints from, on average, 302 with no constraints to 276, 273, 223, 96, and only 13. A similar pattern is observed for other factors, albeit not always monotonic.

Our model is also able to attribute performance differences between integrated and mixed-sleeve multi-factor portfolios to differences in their factor characteristics, and thus resolve the heated discussion in the literature over which approach is better for construction of portfolios with exposures to multiple factors. We further show that return differences between factor portfolios in the small-cap and the large-cap space, and between equally-weighted and value-weighted factor portfolios can also be explained by our model."

Are you looking for more strategies to read about? Check http://quantpedia.com/Screener

Do you want to see performance of trading systems we described? Check http://quantpedia.com/Chart/Performance

Do you want to know more about us? Check http://quantpedia.com/Home/About

Follow us on:

Facebook: https://www.facebook.com/quantpedia/

Twitter: https://twitter.com/quantpedia