Financial academics have described so many equity factors that the whole universe of them is sometimes called “factor zoo”. Therefore, it is no surprise that there is a quest within an academic community to bring some order into this chaos. An interesting research paper written by Favilukis and Zhang suggests explaining a lot of equity factors with momentum anomaly. They show that very often, up to 50% of the equity factor returns can be linked to returns of momentum strategy. This link is especially prevalent in short legs of equity factors .

Authors: Favilukis, Zhang

Title: One Anomaly to Explain Them All

Link: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=3444342

Abstract:

We argue that conditional on the existence of momentum, many other asset pricing anomalies are not particularly anomalous. First, empirically, we show that portfolios within which conditional momentum strategies (ie buying winners and selling losers) are unprofitable, tend to have significantly higher unconditional average returns than portfolios within which momentum strategies are profitable. Second, we rationalize this in a standard model to which we add momentum; the intuition is that assets with more conditional trading opportunities are bid up by speculators and tend to have higher prices and lower unconditional returns. Third, we show that for many asset pricing anomalies, a momentum strategy tends to be unprofitable within the long leg, but profitable within the short leg. Thus, according to our model, the long leg should earn higher unconditional average returns, which explains the anomaly. Once accounting for this effect, the average Fama French 3 factor alpha across 36 prominent anomalies falls by up to 47%. Finally, we show that although the CAPM beta is negatively related to the average unconditional return of a large set of portfolios, it is strongly positively related to the average conditional return of the same set of portfolios, which helps explain the apparent empirical failure of the CAPM.

Notable quotations from the academic research paper:

” We propose an unified explanation for the high excess returns on a large set of anomalies, the explanation relies on the existence of momentum. While our model endogenously generates momentum, our goal is not to explain momentum, rather it is to show that conditional on its existence, the high observed returns on many anomaly portfolios are to be expected. We first sort firms into various portfolios based on 37 prominent anomalies identified in the literature, with ten portfolios per anomaly and 370 total portfolios. For example firms in the bottom decile of the size distribution, or firms in the third decile of the operating profitability distribution are two such portfolios. Within each portfolio, we compute the return from following a momentum strategy, that is buying past winners and selling past losers only within this portfolio. Momentum profits are systematically different across portfolios, for example firms in the top idiosyncratic volatility decile persistently earn high momentum returns, while firms in the bottom idiosyncratic volatility decile persistently earn low momentum returns.

Our first key finding is that there is a strong negative relationship between a portfolio’s momentum profits, and its buy-and-hold return. That is, portfolios that tend to systematically offer momentum trading opportunities (MTOs) and have high conditional trading returns, such as high idiosyncratic volatility firms, tend to also have low unconditional or buy-and-hold returns. Figure 1 illustrates this fact. 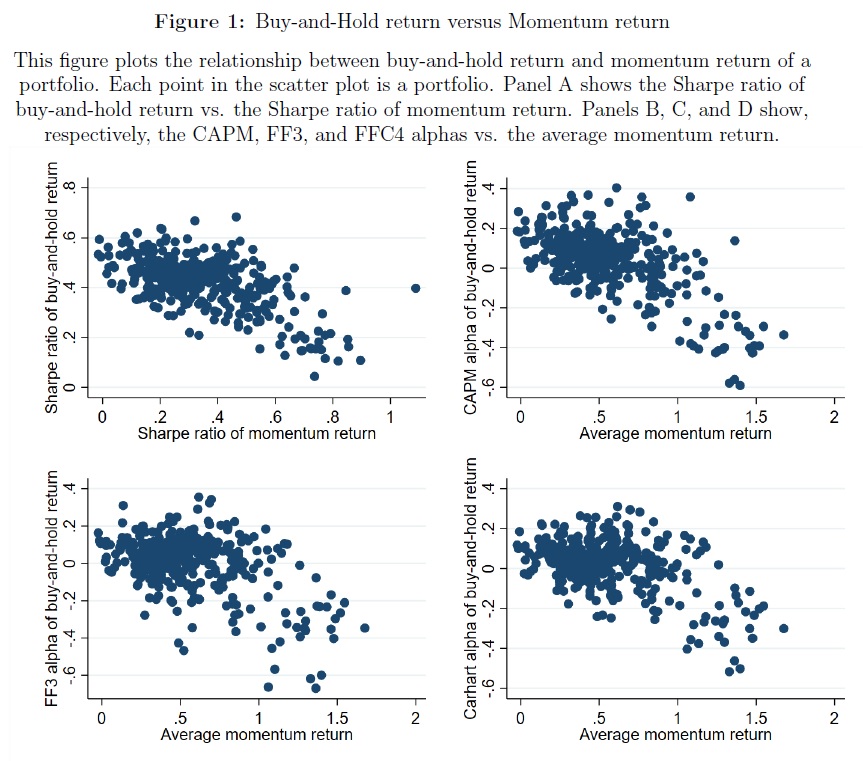Our second key finding is that MTOs tend to be present in the short leg, that is the low buy-and-hold return leg, of many anomalies. For example, high market-to-book, low cash-flow yield, high investment rate, low operating profitability, high idiosyncratic volatility, and high distress firms all offer great MTOs { a momentum strategy within such firms is very profitable. These are also firms which have low average buy-and-hold returns, as identified by the anomaly literature. Thus, we identify a common feature across many seemingly unrelated anomalies. We argue that when one sorts on some characteristic that the literature has identified as leading to high (anomalous) returns, what one is actually sorting on is MTOs. Controlling for the presence of MTOs, the average long-short anomaly alpha is reduced by between 23% and 47%.

Our third key finding is theoretical and underpins the first two. We show that if MTOs are present in a set of firms, then these same firms should have lower average buy-and-hold returns. Thus, through the lens of our model, any anomaly whose short leg offers MTOs should have a positive return spread between its long and short legs. The model’s intuition is that active, or speculative traders are more interested in firms which offer active trading opportunities, than those firms that do not. As a result, MTO firms have higher unconditional prices and lower unconditional expected returns, despite offering high returns for traders who follow an active (conditional) strategy.”

Are you looking for more strategies to read about? Sign up for our newsletter or visit our Blog or Screener.

Do you want to learn more about Quantpedia Premium service? Check how Quantpedia works, our mission and Premium pricing offer.

Do you want to learn more about Quantpedia Pro service? Check its description, watch videos, review reporting capabilities and visit our pricing offer.

Are you looking for historical data or backtesting platforms? Check our list of Algo Trading Discounts.

Or follow us on:

Facebook Group, Facebook Page, Twitter, Linkedin, Medium or Youtube