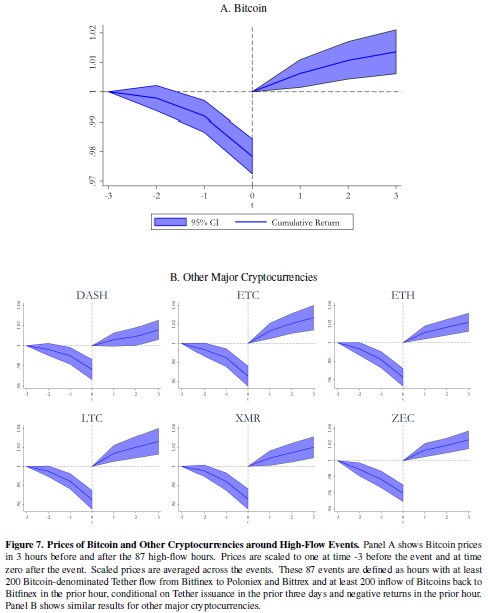
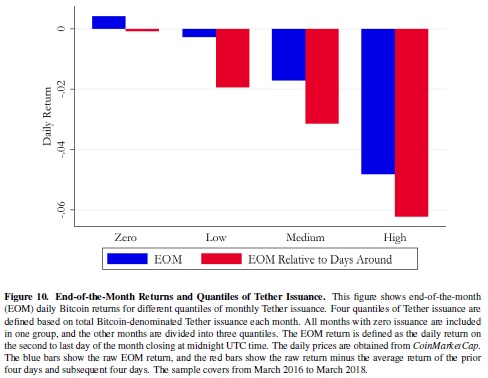
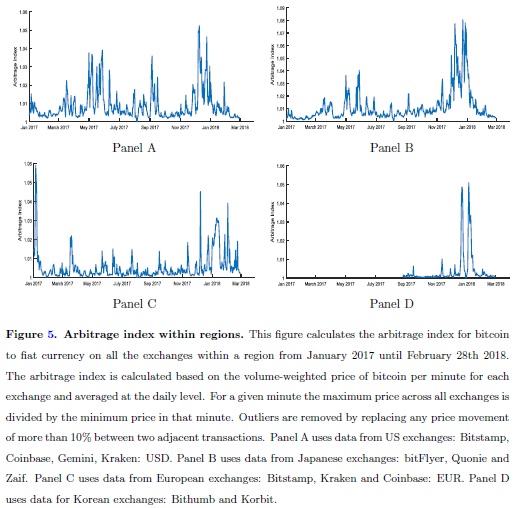
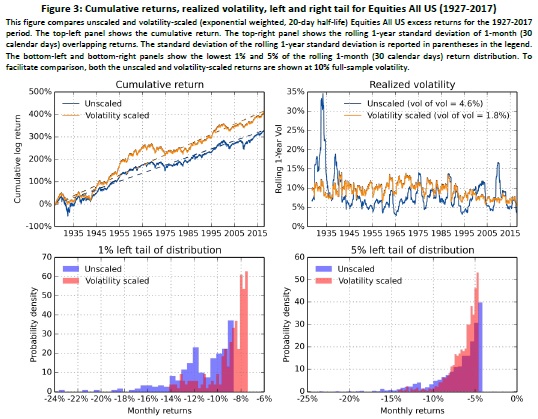
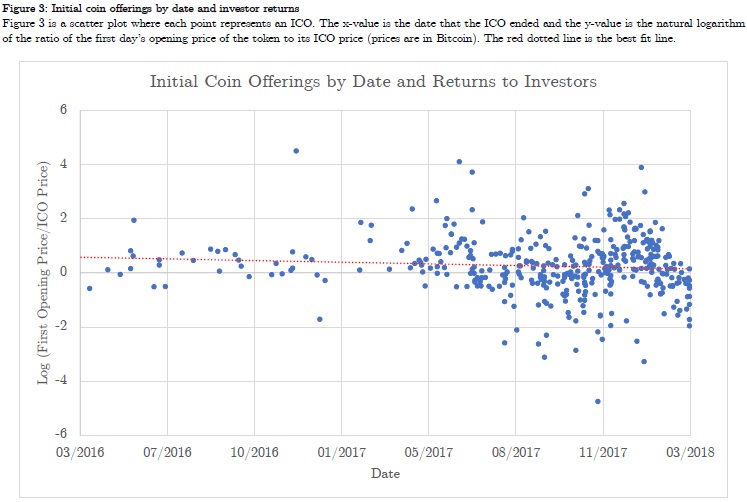
Announcing QuantConnect & Quantpedia Cooperation
The Quantpedia site was created seven years ago and, over time, our pet project evolved into something bigger. We started small, but we always wanted to have a wide scope of strategies in our database. Our goal was to find, analyze, describe and categorize as many quant trading strategies as possible. Thus, we did not limit our focus to only one asset class, trading style, or instrument type.
Our database contains strategies on all asset classes (equities, bonds, commodities, etc.), trading styles, and popular instruments. However, this very broad approach brings one major issue: we’ve never had the resources to backtest the strategies we find in the thousands of academic research papers, so we could never independently validate them. Until now…
I am really excited to introduce our cooperation with QuantConnect. With the company’s resources and extensive database of historical data, combined with a backtesting engine, QuantConnect was able to start systematically backtesting strategies from our database. And now, we can finally start showing you out-of-sample backtests for some of our strategies.
If you’re unfamiliar, QuantConnect is a cloud-based algorithmic trading platform that enables its community of more than 60k quants, computer scientists, and engineers to backtest and deploy live strategies to brokerages such as Interactive Brokers and Coinbase Pro.
At the moment, QuantConnect has covered nearly 20 strategies and will continue to periodically add new strategy implementations in the future.
The QuantConnect out-of-sample backtests can be found at the bottom of Quantpedia strategy's subpage. Let’s look, for example, at strategy #2 – Asset Class Momentum. If you scroll down on that page, after a description of Asset Class Momentum strategy and links to source and related research papers, you’ll see a section with embedded code, an out-of-sample chart, and performance and risk statistics, all from QuantConnect.
Embedded code:

An out-of-sample chart:
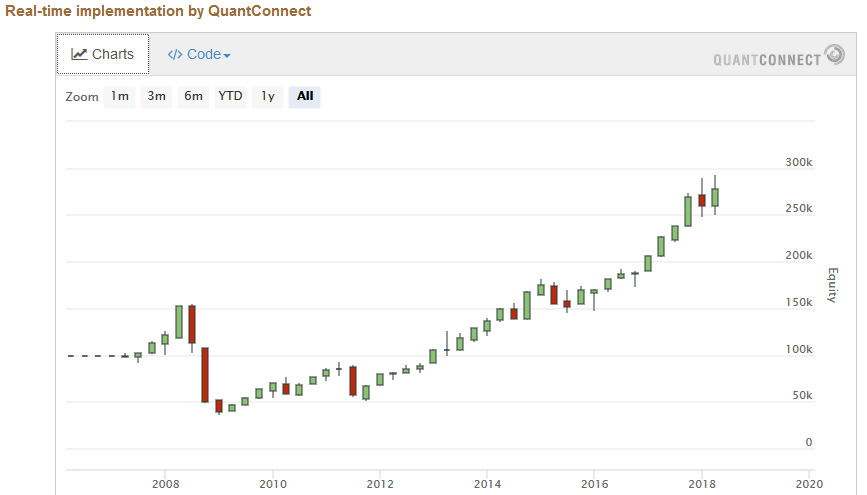
Performance and risk statistics:
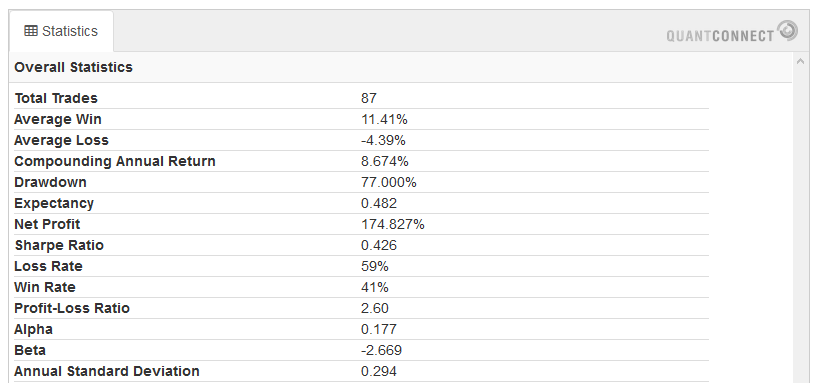
We plan to add a new field into our Screener (https://quantpedia.com/Screener) which will allow you to filter our strategies with QuantConnect backtests. Until then, a list of links to strategies that currently have this feature enabled is below:
#1 – Asset Class Trend Following (+ link to Quantconnect's subpage Asset Class Trend Following)
#2 – Asset Class Momentum (+ link to Quantconnect's subpage Asset Class Momentum)
#3 – Sector Momentum (+ link to Quantconnect's subpage Sector Momentum)
#4 – Overnight Anomaly (+ link to Quantconnect's subpage Overnight Anomaly)
#5 – Forex Carry Trade (+ link to Quantconnect's subpage Forex Carry Trade)
#7 – Volatility Effect in Stocks (+ link to Quantconnect's subpage Volatility Effect in Stocks)
#8 – Forex Momentum (+ link to Quantconnect's subpage Forex Momentum)
#12 – Pairs Trading (+ link to Quantconnect's subpage Pairs Trading – Cupola vs. Cointegration)
#13 – Short Term Reversal (+ link to Quantconnect's subpage Short Term Reversal)
#14 – Momentum Effect in Stocks (+ link to Quantconnect's subpage Momentum Effect in Stocks)
#15 – Momentum Effect in Country Equity Indexes (+ link to Quantconnect's subpage Momentum Effect in Country Equity Indexes)
#16 – Mean Reversion Effect in Country Equity Indexes (+ link to Quantconnect's subpage Mean Reversion Effect in Country Equity Indexes)
#18 – Liquidity Effect in Stocks (+ link to Quantconnect's subpage Liquidity Effect in Stocks)
#20 – Volatility Risk Premium Effect (+ link to Quantconnect's subpage Volatility Risk Premium Effect)
#21 – Momentum Effect in Commodities (+ link to Quantconnect's subpage Momentum Effect in Commodities)
#22 – Term Structure Effect in Commodities (+ link to Quantconnect's subpage Term Structure Effect in Commodities)
#25 – Small Capitalization Stocks Premium Anomaly (+ link to Quantconnect's subpage Small Capitalization Stocks Premium Anomaly)
#26 – Book-to-Market Value Anomaly (+ link to Quantconnect's subpage Book-to-Market Value Anomaly)
We are really thrilled that we can show you this new part of our analysis, and we are already looking forward to backtesting additional strategies with QuantConnect’s engine…
Are you looking for more strategies to read about? Check http://quantpedia.com/Screener
Do you want to see performance of trading systems we described? Check http://quantpedia.com/Chart/Performance
Do you want to know more about us? Check http://quantpedia.com/Home/About
Follow us on:
Facebook: https://www.facebook.com/quantpedia/
Twitter: https://twitter.com/quantpedia